Modern Physics 5 Question 1
1. Half lives of two radioactive nuclei
60 minutes, the ratio of decayed numbers of nuclei
(Main 2019, 12 April II)
(a)
(b)
(c)
(d)
Show Answer
Answer:
Correct Answer: 1. (d)
Solution:
- For substance
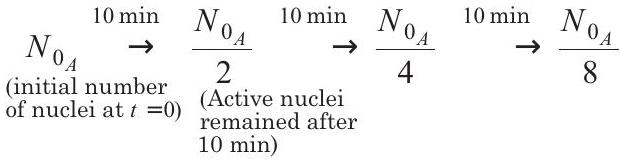
Similarly, for substance
So, number of nuclei of
Hence, ratio of decayed nuclei of
Alternate Solution
Number of active nuclei remained after
where,
So, for nuclei
Similarly, for nuclei






