Magnetics 6 Question 1
1. A moving coil galvanometer, having a resistance $G$, produces full scale deflection when a current $I _g$ flows through it. This galvanometer can be converted into (i) an ammeter of range 0 to $I _0\left(I _0>I _g\right)$ by connecting a shunt resistance $R _A$ to it and (ii) into a voltmeter of range 0 to $V\left(V=G I _0\right)$ by connecting a series resistance $R _V$ to it. Then,
(2019 Main, 12 April II)
(a) $R _A R _V=G^{2} \frac{I _0-I _g}{I _g}$ and $\frac{R _A}{R _V}=\frac{I _g}{\left(I _0-I _g\right)}$
(b) $R _A R _V=G^{2}$ and $\frac{R _A}{R _V}=\frac{I _g}{I _0-I _g}$ (c)
(d)
Show Answer
Solution:
- To use galvanometer as an ammeter, a low resistance in parallel is used.
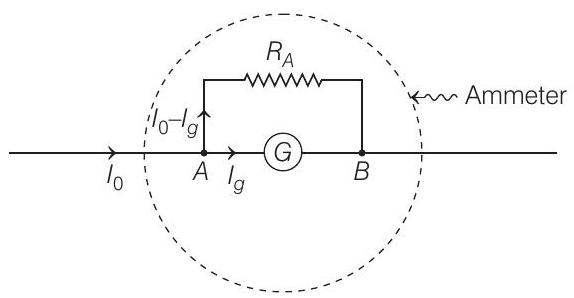
In ammeter, if $I _g=$ full scale deflection current, then equating potential drops across points marked $A B$, we have
$$ V _{A B}=I _g G=\left(I _0-I _g\right) R _A $$
$$ \Rightarrow \quad R _A=\frac{I _g G}{I _0-I _g} $$
Here, $G$ =resistance of galvanometer coil.
When a galvanometer is used as a voltmeter, a high resistance $\left(R _V\right)$ in series is used.
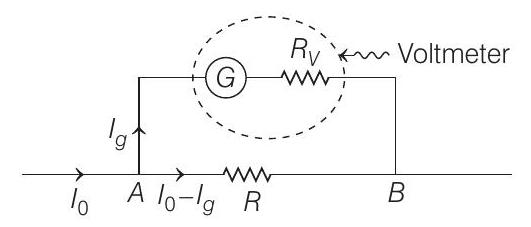
Equating potential across point $A B$,
$$ \begin{array}{lll} & & V _{A B}=\left(G+R _V\right) I _g \\ \text { But } & V _{A B}=I _0 G \\ \text { So, } & I _0 G=\left(G+R _V\right) I _g \\ \Rightarrow & R _V=\frac{\left(I _0-I _g\right) G}{I _g} \end{array} $$
(given)
From Eqs. (i) and (ii), we have
$$ \frac{R _A}{R _V}=\frac{\frac{I _g G}{I _0-I _g}}{\frac{\left(I _0-I _g\right) G}{I _g}}=\frac{I _g^{2}}{\left(I _0-I _g\right)^{2}} $$
and $\quad R _A \times R _V=\frac{I _g G}{\left(I _0-I _g\right)} \times \frac{\left(I _0-I _g\right) G}{I _g}=G^{2}$






