Magnetics 4 Question 6
7. A loop carrying current $I$ lies in the $x-y$ plane as shown in the figure. The unit vector $\hat{\mathbf{k}}$ is coming out of the plane of the paper. The magnetic moment of the current loop is
(2012)
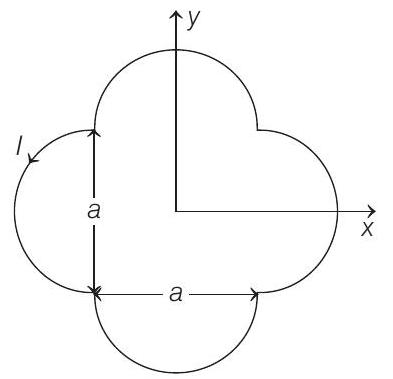
(a) $a^{2} I \hat{\mathbf{k}}$
(b) $\frac{\pi}{2}+1 a^{2} I \hat{\mathbf{k}}$
(c) $-\frac{\pi}{2}+1 a^{2} I \hat{\mathbf{k}}$
(d) $(2 \pi+1) a^{2} I \hat{\mathbf{k}}$
Show Answer
Answer:
Correct Answer: 7. (b)
Solution:
- Area of the given loop is
$A=$ (area of two circles of radius $\frac{a}{2}$ and area of a square of side $a$ )
$$ \begin{aligned} &=2 \pi \frac{a}{2}^{2}+a^{2}=\frac{\pi}{2}+1 a^{2} \\ &|\mathbf{M}|=I A=\frac{\pi}{2}+1 a^{2} I \end{aligned} $$
From screw law direction of $\mathbf{M}$ is outwards or in positive $z$-direction.
$$ \therefore \quad \mathbf{M}=\frac{\pi}{2}+1 a^{2} I \hat{\mathbf{k}} $$






