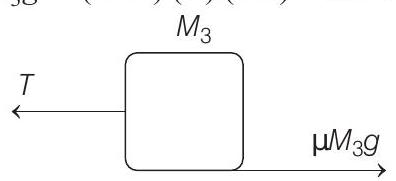
Laws of Motion 3 Question 22
23. Masses
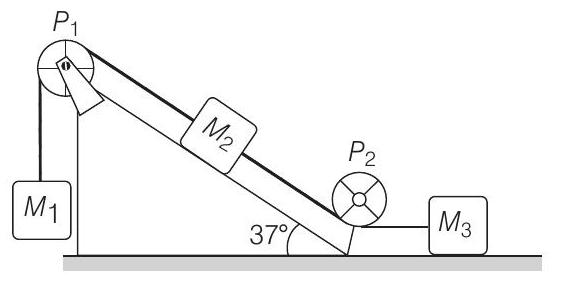
The masses move such that the portion of the string between
If the mass
(1981, 6M)
(a) the mass of
(b) the tension in the horizontal portion of the string.
(Take
Show Answer
Answer:
Correct Answer: 23. (a)
(a)
Solution:
- Constant velocity means net acceleration of the system is zero. Or net pulling force on the system is zero. While calculating the pulling force, tension forces are not taken into consideration. Therefore,
(a)
Substituting the values
(b) Since,