Laws of Motion 2 Question 4
4. The pulleys and strings shown in the figure are smooth and of negligible mass. For the system to remain in equilibrium, the angle
(2001, 2M)
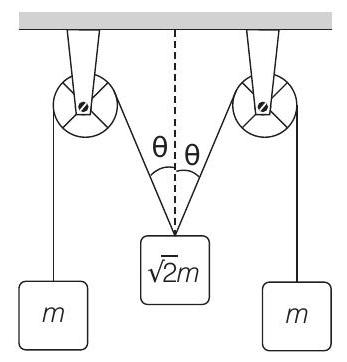
(a)
(b)
(c)
(d)
Show Answer
Answer:
Correct Answer: 4. (d)
Solution:
- Free body diagram of
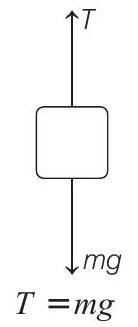
Free body diagram of mass
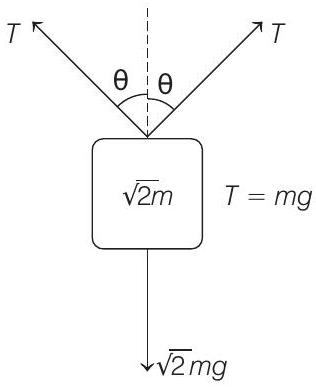
Dividing Eq. (ii) by Eq. (i), we get






