Heat and Thermodynamics 5 Question 20
21. An ideal gas expands isothermally from a volume
(2004, 2M)
(a)
(b)
(c)
(d)
Show Answer
Solution:
- Slope of adiabatic process at a given state
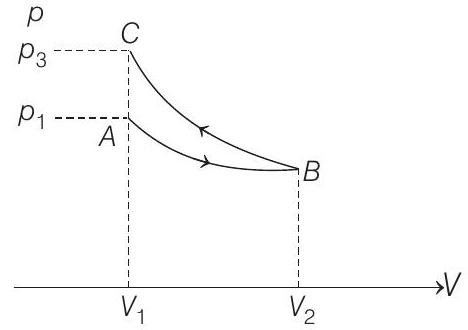
In the graph,
and
Hence,
From the graph itself, it is clear that
NOTE At point






