Heat and Thermodynamics 5 Question 16
17. One mole of diatomic ideal gas undergoes a cyclic process
(2014 Main)
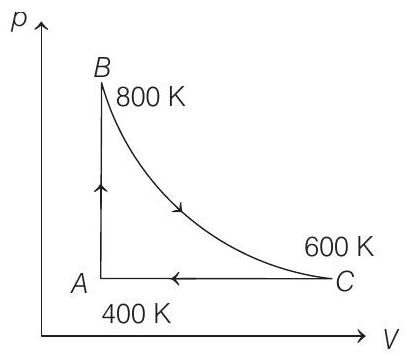
(a) The change in internal energy in whole cyclic process is
(b) The change in internal energy in the process
(c) The change in internal energy in the process
(d) The change in internal energy in the process
Show Answer
Solution:
- According to first law of thermodynamics, we get
(i) Change in internal energy from
(ii) Change in internal energy from
(iii)
(iv) Change in internal energy from






