Heat and Thermodynamics 2 Question 5
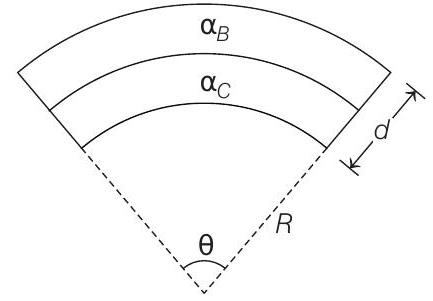
8. A bimetallic strip is formed out of two identical strips-one of copper and the other of brass. The coefficients of linear expansion of the two metals are
(a) proportional to
(b) inversely proportional to
(c) proportional to
(d) inversely proportional to
Integer Answer Type Question
Show Answer
Answer:
Correct Answer: 8.
Solution:
- Let
[From binomial expansion]






