Electrostatics 7 Question 23
25. Four point charges, each of
(2011)
True/False
Show Answer
Solution:
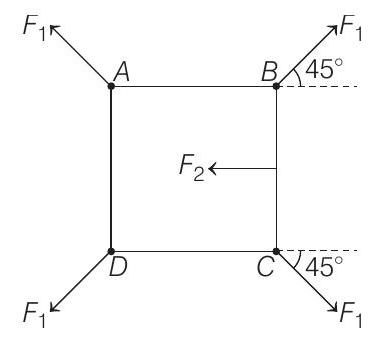
If we see the equilibrium of line
where,
Therefore,
Answer is 3 .






