Electrostatics 7 Question 22
24. A positively charged thin metal ring of radius $R$ is fixed in the $x-y$ plane with its centre at the origin $O$. A negatively charged particle $P$ is released from rest at the point $\left(0,0, z _0\right)$ where $z _0>0$. Then the motion of $P$ is
(a) periodic for all values of $z _0$ satisfying $0<z _0<\infty$
(b) simple harmonic for all values of $z _0$ satisfying $0<z _0 \leq R$
(c) approximately simple harmonic provided $z _0«R$
(d) such that $P$ crosses $O$ and continues to move along the negative $z$-axis towards $z=-\infty$
Integer Answer Type Questions
Show Answer
Solution:
- Let $Q$ be the charge on the ring, the negative charge $-q$ is released from point $P\left(0,0, z _0\right)$. The electric field at $P$ due to the charged ring will be along positive $z$-axis and its magnitude will be
$$ E=\frac{1}{4 \pi \varepsilon _0} \frac{Q z _0}{\left(R^{2}+z _0^{2}\right)^{3 / 2}} $$
$E=0$ at centre of the ring because $z _0=0$
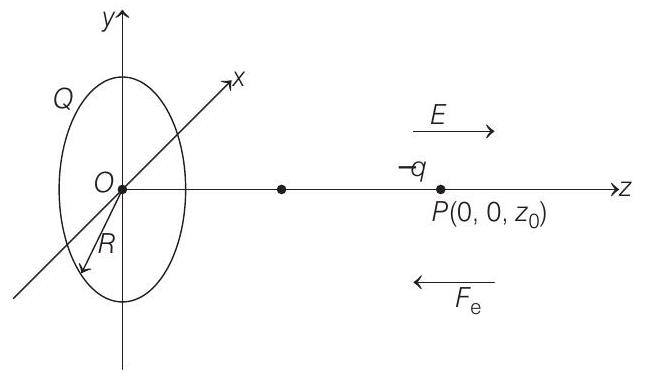
Force on charge at $P$ will be towards centre as shown, and its magnitude is
$$ F _e=q E=\frac{1}{4 \pi \varepsilon _0} \cdot \frac{Q q}{\left(R^{2}+z _0^{2}\right)^{3 / 2}} \cdot z _0 $$
Similarly, when it crosses the origin, the force is again towards centre $O$.
Thus, the motion of the particle is periodic for all values of $z _0$ lying between 0 and $\infty$.
Secondly, if $z _0«R,\left(R^{2}+z _0^{2}\right)^{3 / 2} \approx R^{3}$
$$ \left.F _e \approx \frac{1}{4 \pi \varepsilon _0} \cdot \frac{Q q}{R^{3}} \cdot z _0 \quad \text { [From Eq. (i) }\right] $$
i.e. the restoring force $F _e \propto-z _0$. Hence, the motion of the particle will be simple harmonic. (Here negative sign implies that the force is towards its mean position.)
