Electrostatics 5 Question 7
8. Voltage rating of a parallel plate capacitor is
(Take,
(Main 2019, 8 April I)
(a) 3.8
(b) 8.5
(c) 4.5
(d) 6.2
Show Answer
Answer:
Correct Answer: 8.
(b)
Solution:
- As we know, capacitance of a capacitor filled with dielectric medium,
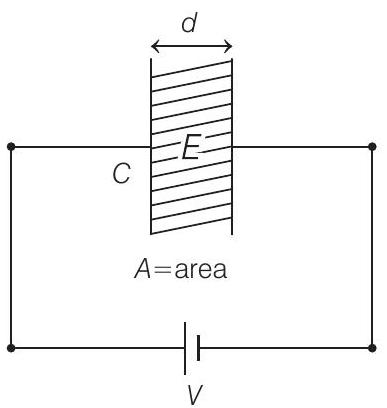
and potential difference between plates is
So, by combining both Eqs. (i) and (ii), we get
Given,
and
Substituting the values in Eq. (iii), we get






