Electrostatics 5 Question 46
48. Two capacitors $A$ and $B$ with capacities $3 \mu F$ and $2 \mu F$ are charged to a potential difference of $100 V$ and $180 V$ respectively. The plates of the capacitors are connected as shown in the figure with one wire of each capacitor free. The upper plate of $A$ is positive and that of $B$ is negative. An uncharged $2 \mu F$ capacitor $C$ with lead wires falls on the free ends to complete the circuit. Calculate
$(1997,5$ M)
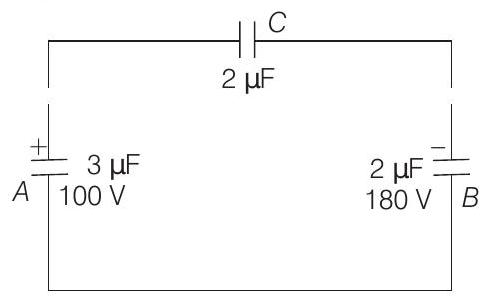
(a) the final charge on the three capacitors and
(b) the amount of electrostatic energy stored in the system before and after completion of the circuit.
Show Answer
Solution:
- (a) Charge on capacitor $A$, before joining with an uncharged capacitor
Similarly, charge on capacitor $B$
$$ q _B=(180)(2) \mu C=360 \mu C $$
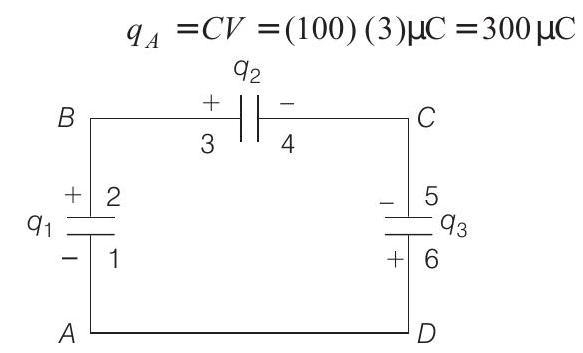
Let $q _1, q _2$ and $q _3$ be the charges on the three capacitors after joining them as shown in figure.
$\left(q _1, q _2\right.$ and $q _3$ are in microcoulombs)
From conservation of charge
Net charge on plates 2 and 3 before joining $=$ net charge after joining
$$ \therefore \quad 300=q _1+q _2 $$
Similarly, net charge on plates 4 and 5 before joining
$=$ net charge after joining $-360=-q _2-q _3$
or $\quad 360=q _2+q _3$
Applying Kirchhoff’s second law in closed loop $A B C D A$
$$ \begin{aligned} \frac{q _1}{3}-\frac{q _2}{2}+\frac{q _3}{2} & =0 \\ \text { or } \quad 2 q _1-3 q _2+3 q _3 & =0 \end{aligned} $$
Solving Eqs. (i), (ii) and (iii), we get
$$ \begin{array}{ll} q _1 & =90 \mu C, q _2=210 \mu C \\ \text { and } \quad q _3 & =150 \mu C \end{array} $$
(b) (i) Electrostatic energy stored before, completing the circuit
$$ \begin{gathered} U _i=\frac{1}{2}\left(3 \times 10^{-6}\right)(100)^{2}+\frac{1}{2}\left(2 \times 10^{-6}\right)(180)^{2} \\ \because U=\frac{1}{2} C V^{2} \\ =4.74 \times 10^{-2} J \text { or } U _i=47.4 mJ \end{gathered} $$
(ii) Electrostatic energy stored after, completing the circuit
$$ \begin{aligned} U _f= & \frac{1}{2} \frac{\left(90 \times 10^{-6}\right)^{2}}{\left(3 \times 10^{-6}\right)}+\frac{1}{2} \frac{\left(210 \times 10^{-6}\right)^{2}}{\left(2 \times 10^{-6}\right)} \\ & +\frac{1}{2} \frac{\left(150 \times 10^{-6}\right)^{2}}{\left(2 \times 10^{-6}\right)} \quad U=\frac{1}{2} \frac{q^{2}}{C} \\ & =1.8 \times 10^{-2} J \quad \text { or } \quad U _f=18 mJ \end{aligned} $$
