Electrostatics 5 Question 33
35. A parallel plate capacitor of capacitance $C$ is connected to a battery and is charged to a potential difference $V$. Another capacitor of capacitance $2 C$ is similarly charged to a potential difference $2 V$. The charging battery is now disconnected and the capacitors are connected in parallel to each other in such a way that the positive terminal of one is connected to the negative terminal of the other. The final energy of the configuration is
$(1995,2 M)$
(a) zero
(b) $\frac{3}{2} C V^{2}$
(c) $\frac{25}{6} C V^{2}$
(d) $\frac{9}{2} C V^{2}$
Show Answer
Solution:
- The diagramatic representation of given problem is shown in figure.
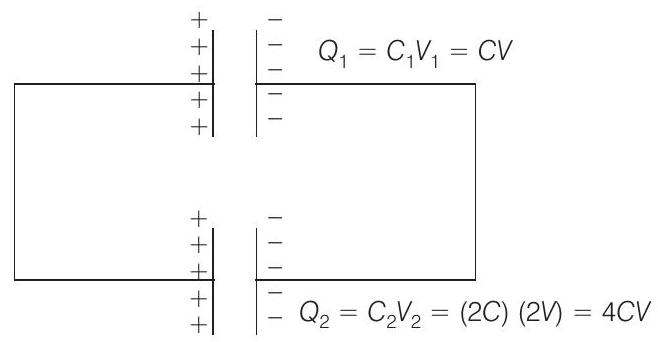
The net charge shared between the two capacitors is
$$ Q^{\prime}=Q _2-Q _1=4 C V-C V=3 C V $$
The two capacitors will have the same potential, say $V^{\prime}$.
The net capacitance of the parallel combination of the two capacitors will be
$$ C^{\prime}=C _1+C _2=C+2 C=3 C $$
The potential difference across the capacitors will be
$$ V^{\prime}=\frac{Q^{\prime}}{C^{\prime}}=\frac{3 C V}{3 C}=V $$
The electrostatic energy of the capacitors will be
$$ U^{\prime}=\frac{1}{2} C^{\prime} V^{\prime 2}=\frac{1}{2}(3 C) V^{2}=\frac{3}{2} C V^{2} $$
