Electrostatics 5 Question 20
22. In the given circuit, charge $Q _2$ on the $2 \mu F$ capacitor changes as $C$ is varied from $1 \mu F$ to $3 \mu F . Q _2$ as a function of $C$ is given properly by (figures are drawn schematically and are not to scale)
(2015 Main)
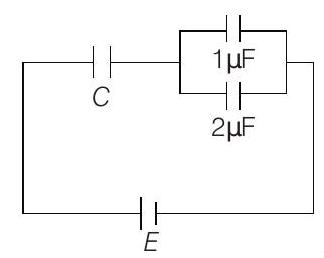
(a)

(c)
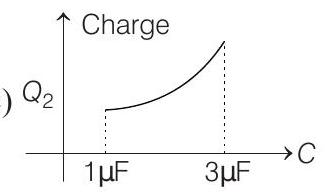
(b)
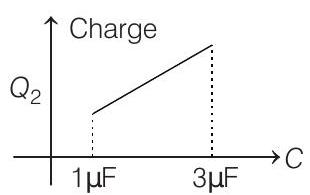
(d)
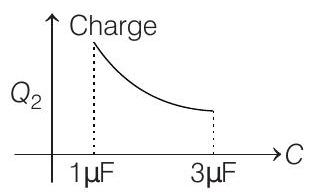
Show Answer
Solution:
- Resultant of $1 \mu F$ and $2 \mu F$ is $3 \mu F$. Now in series, potential difference distributes in inverse ratio of capacity.
$\therefore \quad \frac{V _{3 \mu F}}{V _c}=\frac{c}{3} \quad$ or $\quad V _{3 \mu F}=\frac{c}{c+3} E$
This is also the potential difference across $2 \mu F$.
$$ \begin{array}{ll} \therefore & Q _2=(2 \mu F)\left(V _{2 \mu F}\right) \\ \text { or } & Q _2=\frac{2 c E}{c+3}=\frac{2}{1+\frac{3}{c}} E \end{array} $$
From this expression of $Q _2$, we can see that $Q _2$ will increase with increase in the value of $c$ (but not linearly). Therefore, only options (a) and (b) may be correct.
Further, $\frac{d}{d c}\left(Q _2\right)=2 E \frac{(c+3)-c}{(c+3)^{2}}=\frac{6 E}{(c+3)^{2}}$
$=$ Slope of $Q _2$ verus $c$ graph
i.e. slope of $Q _2$ versus $c$ graph decreases with increase in the value of $c$. Hence, the correct graph is (a).






