Electrostatics 4 Question 5
5. Two electric dipoles, $A, B$ with respective dipole moments $\mathbf{d} _A=-4 q a \hat{\mathbf{i}}$ and $\mathbf{d} _B=-2 q a \hat{\mathbf{i}}$ are placed on the $X$-axis with a separation $R$, as shown in the figure
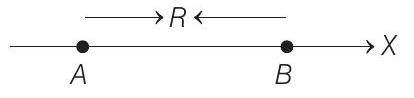
The distance from $A$ at which both of them produce the same potential is
(a) $\frac{\sqrt{2} R}{\sqrt{2}+1}$
(b) $\frac{\sqrt{2} R}{\sqrt{2}-1}$
(c) $\frac{R}{\sqrt{2}+1}$
(d) $\frac{R}{\sqrt{2}-1}$
Show Answer
Solution:
- Key Idea As, dipole moments points in same direction

So, potential of both dipoles can be same at some point between $A$ and $B$. Let potentials are same at $P$, distant $x$ from $B$ as shown below
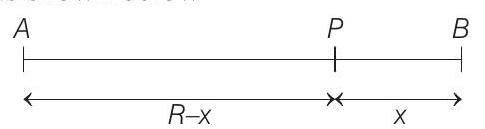
Then,
$$ \begin{aligned} \frac{4 q a}{(R-x)^{2}} & =\frac{2 q a}{(x)^{2}} \\ 2 x^{2} & =(R-x)^{2} \\ \sqrt{2} x & =R-x \Rightarrow x=\frac{R}{\sqrt{2}+1} \end{aligned} $$
Distance from $A$ is
$$ \Rightarrow \quad R-x=R-\frac{R}{\sqrt{2}+1}=\frac{\sqrt{2} R}{\sqrt{2}+1} $$






