Electrostatics 3 Question 13
13. An infinitely long thin non-conducting wire is parallel to the
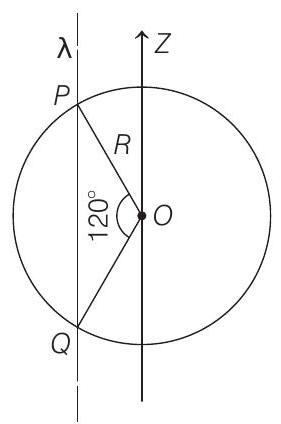
(a) The electric flux through the shell is
(b) The
(c) The electric flux through the shell is
(d) The electric field is normal to the surface of the shell at all points
Show Answer
Answer:
Correct Answer: 13. (c, d)
Solution:
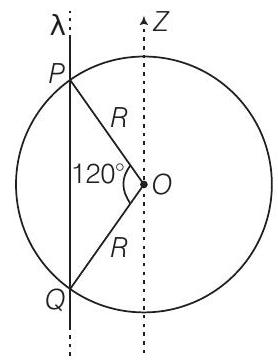
Also, electric field is perpendicular to wire, so






