Electrostatics 1 Question 16
17. A non-conducting solid sphere of radius
(1998, 2M)
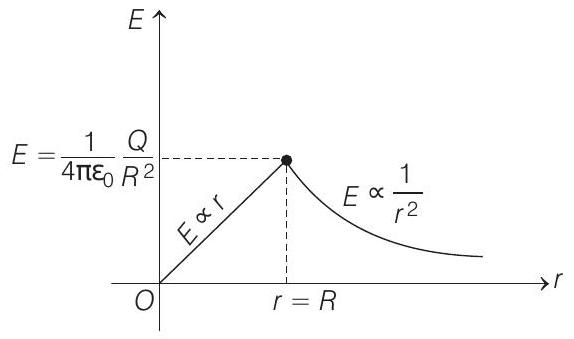
(a) increases as
(b) decreases as
(c) decreases as
(d) is discontinuous at
Show Answer
Answer:
Correct Answer: 17. (c)
Solution:
- Inside the sphere
i.e.
Outside the sphere
or
Thus, variation of electric field