Electromagnetic Induction and Alternating Current 7 Question 12
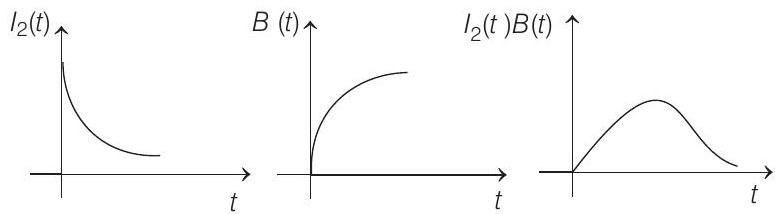
12. A coil of wire having finite inductance and resistance has a conducting ring placed co-axially within it. The coil is connected to a battery at time
(a) increases with time
(b) decreases with time
(c) does not vary with time
(d) passes through a maximum
Show Answer
Answer:
Correct Answer: 12. (d)
Solution:
- The equations of
Therefore, the product