Electromagnetic Induction and Alternating Current 3 Question 4
####4. A copper wire is wound on a wooden frame, whose shape is that of an equilateral triangle. If the linear dimension of each side of the frame is increased by a factor of 3 , keeping the number of turns of the coil per unit length of the frame the same, then the self-inductance of the coil
(Main 2019, 11 Jan II)
(a) increases by a factor of 3 .
(b) decreases by a factor of
(c) increases by a factor of 27 .
(d) decreases by a factor of 9 .
Show Answer
Answer:
Correct Answer: 4. (c)
Solution:
- Self-inductance of a coil is given by the relation
where,
(where,
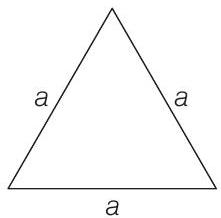
Here,
When each side of frame is increased by a factor 3 keeping the number of turns per unit length of the frame constant.
Then,






