Electromagnetic Induction and Alternating Current 2 Question 8
9. A pair of parallel horizontal conducting rails of negligible resistance shorted at one end is fixed on a table. The distance between the rails is
(1997, 5M)
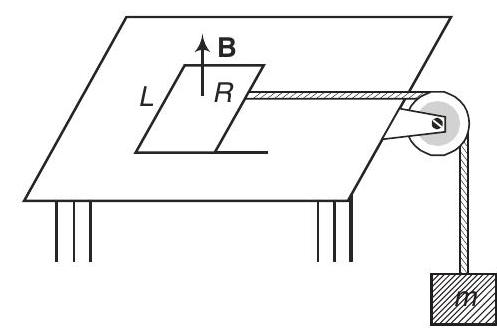
(a) the terminal velocity achieved by the rod, and
(b) the acceleration of the mass at the instant when the velocity of the rod is half the terminal velocity.
Show Answer
Answer:
Correct Answer: 9. (a)
Solution:
- (a) Let
Motional emf,
Motional current,
and magnetic force on the wire
Net force in the system at this moment will be
Velocity will acquire its terminal value i.e.
(b) When
Then from Eq. (i), acceleration of the block,
or






