Electromagnetic Induction and Alternating Current 1 Question 15
15. A rectangular frame
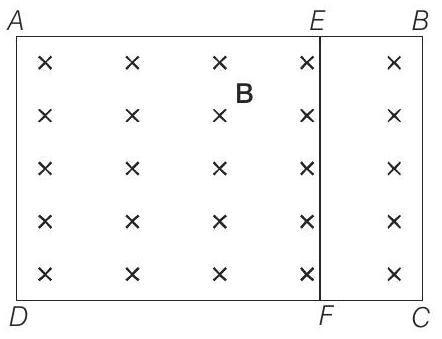
The rate of change of the magnetic field is
(1993, 5M)
Show Answer
Answer:
Correct Answer: 15.
Solution:
- Induced emf in two loops
Similarly,
Now, since the magnetic field is increasing, the induced current will produce the magnetic field in
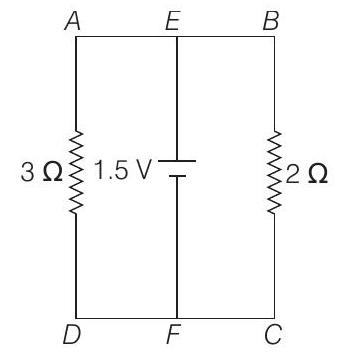
Kirchhoff’s first law at junction
Kirchhoff’s second law in loop FEADF gives
Kirchhoff’s second law in loop
Solving Eqs. (i), (ii) and (iii), we get
Therefore, current in segment






