SATHEE: Current Electricity 2 Question 20

Current Electricity 2 Question 20

20. In the circuit shown below, the key is pressed at time $t = 0$ . Which of the following statement(s) is (are) true?

(2016 Adv.)

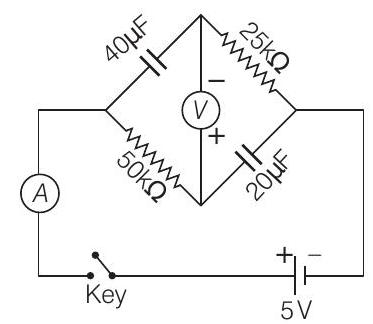
(a) The voltmeter display $- 5 V$ as soon as the key is pressed and displays $+ 5 V$ after a long time

(b) The voltmeter will display $0 V$ at time $t = \ln 2$ seconds

(d) The current in the ammeter becomes zero after a long time

Show Answer

Solution:

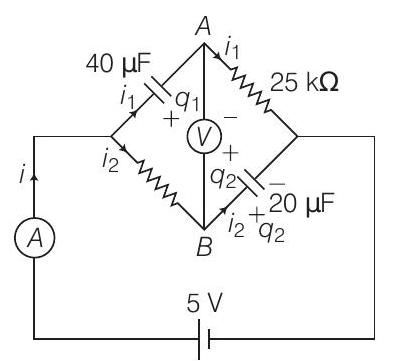
Just after pressing key,

$\begin{aligned} 5 - 25000 i_{1} = 0 \\ 5 - 50000 i_{2} = 0 \end{aligned}$

(As charge in both capacitors)

$\begin{aligned} \Rightarrow i_{1} = 0.2 m A \Rightarrow i_{2} - 0.1 m A \\ And V_{B} + 25000 i_{1} = V_{A} \\ \Rightarrow V_{B} - V_{A} = - 5 V \end{aligned}$

After a long time, $i_{1}$ and $i_{2} = 0$ (steady state)

$\begin{aligned} \Rightarrow & 5 - \frac{q_{1}}{40} & = 0 \\ \Rightarrow & q_{1} & = 200 μ C \end{aligned}$

$\begin{aligned} 5 - \frac{q_{2}}{20} & = 0 \Rightarrow q_{2} = 100 μ C \\ V_{B} - \frac{q_{2}}{20} & = V_{A} \\ \Rightarrow V_{B} - V_{A} & = + 5 V \end{aligned}$

$\Rightarrow$ Option (a) is correct.

For capacitor 1, $q_{1} = 200 [1 - e^{- t / 1}] μ C$

$i_{1} = \frac{1}{5} e^{- t / 1} m A$

For capacitor $2, q_{2} = 100 [1 - e^{- t / 1}] μ C$

$\begin{matrix} i_{2} = \frac{1}{10} e^{- t / 1} m A \\ \Rightarrow V_{B} - \frac{q_{2}}{20} + i_{1} \times 25 = V_{A} \\ \Rightarrow V_{B} - V_{A} = 5 [1 - e^{- t}] - 5 e^{- t} = - 5 [1 - 2 e^{- t}] \\ At t = \ln 2, V_{B} - V_{A} = 5 [1 - 1] = 0 \end{matrix}$