Current Electricity 2 Question 2
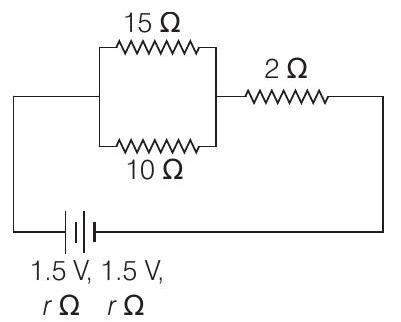
2. In the given circuit, an ideal voltmeter connected across the $10 \Omega$ resistance reads $2 V$. The internal resistance $r$, of each cell is
(2019 Main, 10 April I)
(a) $1.5 \Omega$
(b) $0.5 \Omega$
(c) $1 \Omega$
(d) $0 \Omega$
Show Answer
Solution:
- For the given circuit
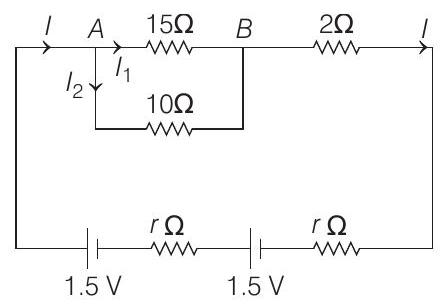
Given,
$$ V _{A B}=2 V $$
$\therefore$ Current in circuit,
$$ \begin{array}{rlr} I=I _1+I _2 & =\frac{2}{15}+\frac{2}{10} \quad[\because V=I R \text { or } I=V / R] \\ & =\frac{4+6}{30}=\frac{1}{3} A \end{array} $$
Also, voltage drop across $(r+r)$ resistors is
= voltage of the cell -voltage drop across $A B$
$$ =3-2=1 V $$
Using $V=I R$ over the entire circuit
$$ \begin{array}{ll} \Rightarrow & 1=I(2+2 r)=\frac{1}{3}(2+2 r) \quad \text { [using Eq. (i)] } \\ \Rightarrow & 3=2+2 r \text { or } 2 r=1 \Omega \\ \text { or } & r=\frac{1}{2} \Omega=0.5 \Omega \end{array} $$
Alternative Solution
Equivalent resistance between $A$ and $B$ is
$$ \frac{1}{R _{A B}}=\frac{1}{10}+\frac{1}{15}=\frac{1}{6} \Rightarrow R _{A B}=6 \Omega $$
$\therefore$ Equivalent resistance of the entire circuit is, $R _{eq}$
$$ =6 \Omega+2 \Omega+2 r=8+2 r $$
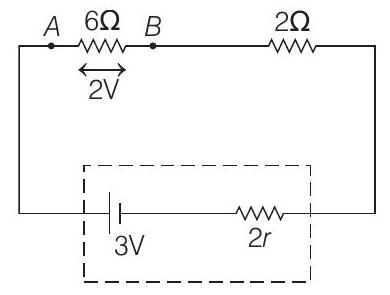
Now, current passing through the circuit is given as,
$$ I=\frac{E _{net}}{R+r _{eq}}=\frac{E _{net}}{R _{eq}} $$
where, $R$ is external resistance, $r _{eq}$ is net internal resistance and $E _{\text {net }}$ is the emf of the cells.
$$ \begin{aligned} & \text { Here, } \quad E _{\text {net }}=1.5+1.5=3 V \\ & r _{eq}=r+r=2 r \\ & \Rightarrow \quad I=\frac{3}{8+2 r} \end{aligned} $$
Also, reading of the voltmeter, $V=2 V=I \cdot R _{A B}$
$$ \begin{aligned} 2 & =\frac{3}{8+2 r} \times 6 \\ \Rightarrow \quad 8+2 r & =9 \text { or } \quad r=\frac{1}{2}=0.5 \Omega \end{aligned} $$
