Centre of Mass 3 Question 35
38. A
(1979)
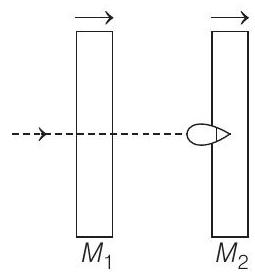
Show Answer
Answer:
Correct Answer: 38.
Solution:
- Applying conservation of linear momentum twice. We have
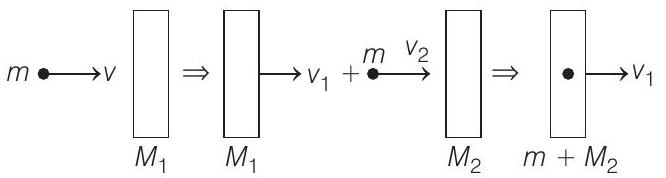
Solving Eqs. (i) and (ii), we get
Substituting the values of






