Centre of Mass 3 Question 2
2. Two particles of masses $M$ and $2 M$, moving as shown, with speeds of $10 m / s$ and $5 m / s$, collide elastically at the origin. After the collision, they move along the indicated directions with speed $v _1$ and $v _2$ are nearly
(2019 Main, 10 April I)
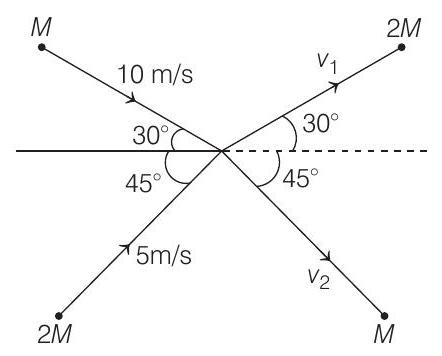
(a) $6.5 m / s$ and $3.2 m / s$
(b) $3.2 m / s$ and $6.3 m / s$
(c) $3.2 m / s$ and $12.6 m / s$
(d) $6.5 m / s$ and $6.3 m / s$
Show Answer
Answer:
Correct Answer: 2. (d)
Solution:
- The given condition can be drawn as shown below
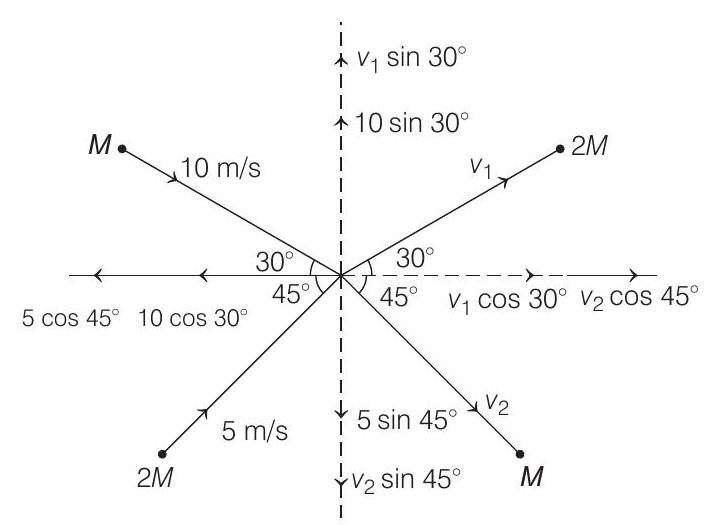
Applying linear momentum conservation law in $x$-direction, we get
Initial momentum $=$ Final momentum
$$ \begin{aligned} &\left(M \times 10 \cos 30^{\circ}\right)+\left(2 M \times 5 \cos 45^{\circ}\right) \\ &=\left(M \times v _2 \cos 45^{\circ}\right)+\left(2 M \times v _1 \cos 30^{\circ}\right) \\ & \Rightarrow \quad M \times 10 \times \frac{\sqrt{3}}{2}+2 M \times 5 \times \frac{1}{\sqrt{2}} \\ &= M \times v _2 \times \frac{1}{\sqrt{2}}+2 M \times v _1 \times \frac{\sqrt{3}}{2} \\ & \Rightarrow \quad 5 \sqrt{3}+5 \sqrt{2}=\frac{v _2}{\sqrt{2}}+v _1 \sqrt{3} \quad \ldots \text { (i) } \end{aligned} $$
Similarly, applying linear momentum conservation law in $y$-direction, we get
$\left(M \times 10 \sin 30^{\circ}\right)-\left(2 M \times 5 \sin 45^{\circ}\right)$
$=\left(M \times v _2 \sin 45^{\circ}\right)-\left(2 M \times v _1 \sin 30^{\circ}\right)$
$\Rightarrow M \times 10 \times \frac{1}{2}-2 M \times 5 \times \frac{1}{\sqrt{2}}$
$=M \times v _2 \times \frac{1}{\sqrt{2}}-2 M \times v _1 \times \frac{1}{2}$
$$ \Rightarrow \quad 5-5 \sqrt{2}=\frac{v _2}{\sqrt{2}}-v _1 $$
Subtracting Eq. (ii) from Eq. (i), we get
$$ \begin{aligned} & (5 \sqrt{3}+5 \sqrt{2})-(5-5 \sqrt{2}) \\ & =\frac{v _2}{\sqrt{2}}+v _1 \sqrt{3}-\frac{v _2}{\sqrt{2}}-v _1 \\ & \Rightarrow \quad 5 \sqrt{3}+10 \sqrt{2}-5=v _1 \sqrt{3}+v _1 \\ & \Rightarrow \quad v _1=\frac{5 \sqrt{3}+10 \sqrt{2}-5}{1+\sqrt{3}}=\frac{8.66+14.142-5}{1+1.732} \\ & =\frac{17.802}{2.732} \Rightarrow v _1=6.516 m / s \approx 6.5 m / s \\ & \text {… (iii) } \end{aligned} $$
Substituting the value from Eq. (iii) in Eq. (i), we get
$$ \begin{array}{rlrl} 5 \sqrt{3}+5 \sqrt{2} & =\frac{v _2}{\sqrt{2}}+6.51 \times \sqrt{3} \\ \Rightarrow \quad & v _2 & =(5 \sqrt{3}+5 \sqrt{2}-6.51 \times \sqrt{3}) \sqrt{2} \\ & & v _2 & =(8.66+7.071-11.215) 1.414 \\ \Rightarrow & v _2 & =4.456 \times 1.414 \\ v _2 & \approx 6.3 m / s \end{array} $$
