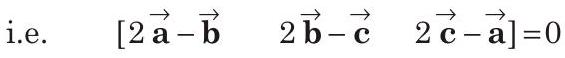Vectors 3 Question 9
9. If
(2000, 2M)
(a) 0
(b) 1
(c)
(d)
Show Answer
Answer:
Correct Answer: 9. (a)
Solution:
- If