Straight Line and Pair of Straight Lines 1 Question 70
70. For a point $P$ in the plane, let $d _1(P)$ and $d _2(P)$ be the distances of the point $P$ from the lines $x-y=0$ and $x+y=0$, respectively. The area of the region $R$ consisting of all points $P$ lying in the first quadrant of the plane and satisfying $2 \leq d _1(P)+d _2(P) \leq 4$, is
(2014 Adv.)
Show Answer
Solution:
- PLAN Distance of a point $\left(x _1, y _1\right)$ from $a x+b y+c=0$ is given by
$$ \left|\frac{a x _1+b y _1+c}{\sqrt{a^{2}+b^{2}}}\right| $$
Let $P(x, y)$ is the point in first quadrant.
Now, $2 \leq\left|\frac{x-y}{\sqrt{2}}\right|+\left|\frac{x+y}{\sqrt{2}}\right| \leq 4$
$$ 2 \sqrt{2} \leq|x-y|+|x+y| \leq 4 \sqrt{2} $$
Case I $x \geq y$
$$ 2 \sqrt{2} \leq(x-y)+(x+y) \leq 4 \sqrt{2} \quad \Rightarrow \quad x \in[\sqrt{2}, 2 \sqrt{2}] $$
Case II $x<y$
$$ 2 \sqrt{2} \leq y-x+(x+y) \leq 4 \sqrt{2} $$
$$ y \in[\sqrt{2}, 2 \sqrt{2}] $$
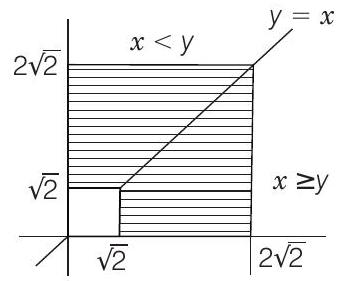
$\Rightarrow A=(2 \sqrt{2})^{2}-(\sqrt{2})^{2}=6$ sq units
