Straight Line and Pair of Straight Lines 1 Question 62
62. One of the diameters of the circle circumscribing the rectangle
(1985, 3M)
Show Answer
Solution:
- Let
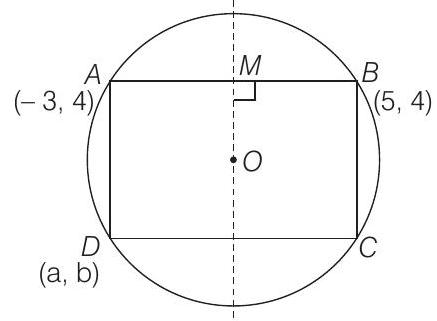
Then,
Since, slope of
Equation of straight line
and
Thus, area of rectangle






