Properties of Triangles 3 Question 11
11. A straight line through the vertex
(a)
(b)
(c)
(d)
Fill in the Blanks
Show Answer
Answer:
Correct Answer: 11. (b, d)
Solution:
- Let a straight line through the vertex
Points
Now,
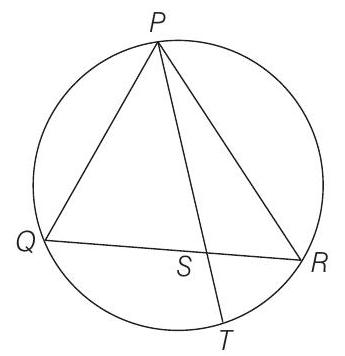
and
Also,






