Properties of Triangles 2 Question 4
4. The sides of a triangle are in the ratio $1: \sqrt{3}: 2$, then the angles of the triangle are in the ratio $(2004,1 M)$
(a) $1: 3: 5$
(b) $2: 3: 2$
(c) $3: 2: 1$
(d) $1: 2: 3$
Fill in the Blank
Show Answer
Answer:
Correct Answer: 4. (d)
Solution:
- Let $a: b: c=1: \sqrt{3}: 2 \Rightarrow c^{2}=a^{2}+b^{2}$
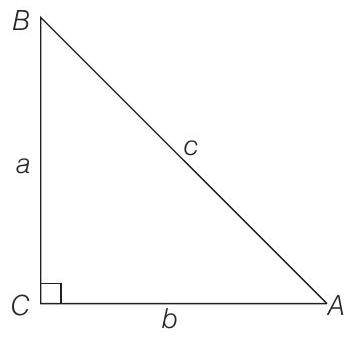
$\therefore$ Triangle is right angled at $C$.
or
$$ \angle C=90^{\circ} $$
and
$$ \frac{a}{b}=\frac{1}{\sqrt{3}} $$
In $\triangle B A C, \quad \tan A=\frac{a}{b}=\frac{1}{\sqrt{3}}$
$$ \Rightarrow \quad A=30^{\circ} $$
and $B=60^{\circ}$
$\left[\because A+B=90^{\circ}\right]$
$\therefore \quad$ Ratio of angles, $A: B: C=30^{\circ}: 60^{\circ}: 90^{\circ}=1: 2: 3$
