Properties of Triangles 1 Question 23
23. $A B C$ is a triangle. $D$ is the middle point of $B C$. If $A D$ is perpendicular to $A C$, then prove that
$$ \cos A \cos C=\frac{2\left(c^{2}-a^{2}\right)}{3 a c} $$
$(1980,3 M)$
Show Answer
Solution:
- In $\triangle A D C$, we have
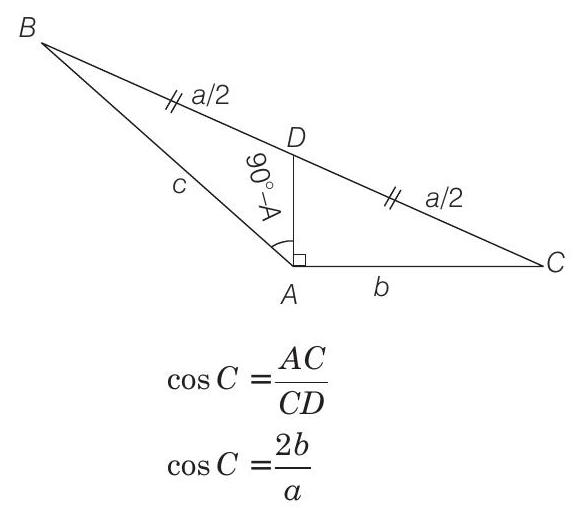
Applying cosine formula in $\triangle A B C$, we have
$$ \begin{aligned} & \cos A=\frac{b^{2}+c^{2}-a^{2}}{2 b c} \\ & \cos C=\frac{a^{2}+b^{2}-c^{2}}{2 a b} \end{aligned} $$
From Eqs. (i) and (ii),
$$ \begin{array}{rlrl} & & \frac{a^{2}+b^{2}-c^{2}}{2 a b} & =\frac{2 b}{a} \\ \Rightarrow & a^{2}+b^{2}-c^{2} & =4 b^{2} \\ \Rightarrow & a^{2}-c^{2} & =3 b^{2} \end{array} $$
Now, $\cos A \cos C=\frac{b^{2}+c^{2}-a^{2}}{2 b c} \cdot \frac{2 b}{a}$
$$ \begin{aligned} & =\frac{b^{2}+c^{2}-a^{2}}{a c}=\frac{3 b^{2}+3\left(c^{2}-a^{2}\right)}{3 a c} \\ & =\frac{\left(a^{2}-c^{2}\right)+3\left(c^{2}-a^{2}\right)}{3 a c}=\frac{2\left(c^{2}-a^{2}\right)}{3 a c} \end{aligned} $$
