SATHEE: Properties of Triangles 1 Question 11

Properties of Triangles 1 Question 11

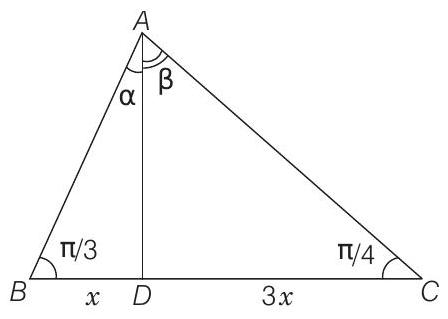
11. In a $△ A B C, ∠ B = \frac{π}{3}$ and $∠ C = \frac{π}{4}$ . Let $D$ divides $B C$ internally in the ratio $1 : 3$ , then $\frac{\sin ∠ B A D}{\sin ∠ C A D}$ is equal to

(a) $\frac{1}{\sqrt{6}}$

(b) $\frac{1}{3}$

(d) $\sqrt{\frac{2}{3}}$

(1995, 2M)

Objective Questions II

(One or more than one correct option)

Show Answer

Answer:

Correct Answer: 11. $(a, b, c, d)$

Solution:

In $△ A B D$ , applying sine rule, we get

$\Rightarrow \begin{aligned} \frac{A D}{\sin π / 3} & = \frac{x}{\sin α} \\ A D & = \frac{\sqrt{3}}{2} x \sin α \end{aligned}$

and in $△ A C D$ , applying sine rule, we get

$\begin{aligned} \frac{A D}{\sin π / 4} & = \frac{3 x}{\sin β} \\ A D & = \frac{3}{\sqrt{2}} x \sin β \end{aligned}$

From Eqs. (i) and (ii), $\frac{\sqrt{3} x}{2 \sin α} = \frac{3 x}{\sqrt{2} \sin β}$

$\Rightarrow \frac{\sin α}{\sin β} = \frac{1}{\sqrt{6}}$

पिछला

अगला

Properties of Triangles 1 Question 11

11. In a $△ A B C, ∠ B = \frac{π}{3}$ and $∠ C = \frac{π}{4}$ . Let $D$ divides $B C$ internally in the ratio $1 : 3$ , then $\frac{\sin ∠ B A D}{\sin ∠ C A D}$ is equal to

Objective Questions II

Answer:

इंजीनियरिंग की तैयारी

चिकित्सा तैयारी

एनसीईआरटी पुस्तकें और समाधान

महत्वपूर्ण संसाधन

अन्य संसाधन

पाठ्यक्रम

परीक्षा मंच

नमूना मॉक टेस्ट

सदस्यता

एनसीईआरटी व्यायाम वीडियो समाधान

Properties of Triangles 1 Question 11

11. In a △ABC,∠B=π3 and ∠C=π4. Let D divides BC internally in the ratio 1:3, then sin⁡∠BADsin⁡∠CAD is equal to

Objective Questions II

Answer:

इंजीनियरिंग की तैयारी

चिकित्सा तैयारी

एनसीईआरटी पुस्तकें और समाधान

महत्वपूर्ण संसाधन

अन्य संसाधन

पाठ्यक्रम

परीक्षा मंच

नमूना मॉक टेस्ट

सदस्यता

एनसीईआरटी व्यायाम वीडियो समाधान

Menu

11. In a $△ A B C, ∠ B = \frac{π}{3}$ and $∠ C = \frac{π}{4}$ . Let $D$ divides $B C$ internally in the ratio $1 : 3$ , then $\frac{\sin ∠ B A D}{\sin ∠ C A D}$ is equal to