Permutations and Combinations 2 Question 24
24.
(1982, 5M)
Show Answer
Solution:
- Let
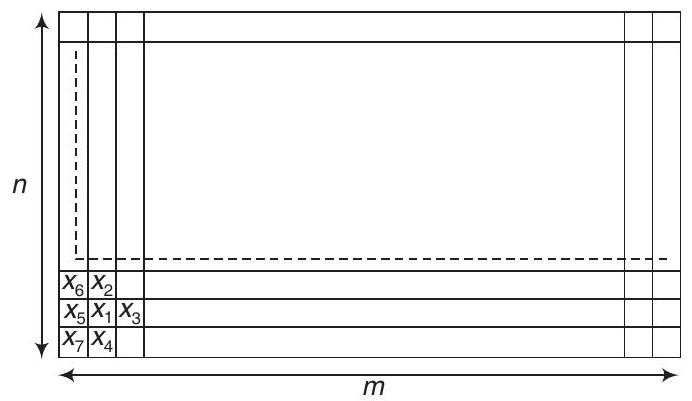
neighbours of
where,






