Inverse Circular Functions 2 Question 15
15. If $f:[0,4 \pi] \rightarrow[0, \pi]$ be defined by $f(x)=\cos ^{-1}(\cos x)$. Then, the number of points $x \in[0,4 \pi]$ satisfying the equation $f(x)=\frac{10-x}{10}$, is
(2014 Adv.)
Show Answer
Solution:
- PLAN
(i) Using definition of $f(x)=\cos ^{-1}(x)$, we trace the curve $f(x)=\cos ^{-1}(\cos x)$
(ii) The number of solutions of equations involving trigonometric and algebraic functions and involving both functions are found using graphs of the curves.
We know that, $\cos ^{-1}(\cos x)=\begin{array}{ll}x, & \text { if } x \in[0, \pi] \ 2 \pi-x, & \text { if } x \in[\pi, 2 \pi] \ -2 \pi+x, & \text { if } x \in[2 \pi, 3 \pi] \ 4 \pi-x, & \text { if } x \in[3 \pi, 4 \pi]\end{array}$
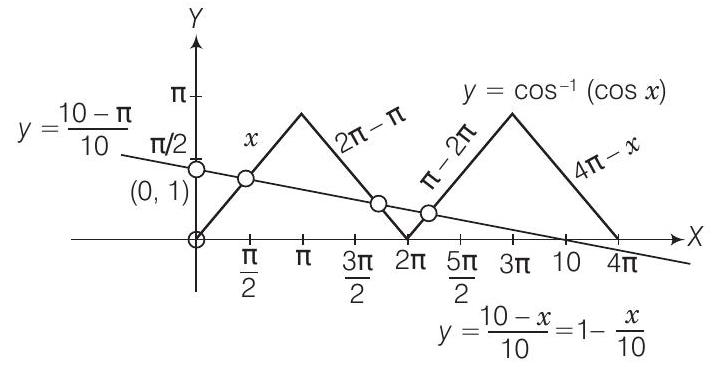
From above graph, it is clear that $y=\frac{10-x}{10}$ and $y=\cos ^{-1}(\cos x)$ intersect at three distinct points, so number of solutions is 3 .
