Hyperbola 1 Question 16
17. A variable straight line of slope 4 intersects the hyperbola $x y=1$ at two points. Find the locus of the point which divides the line segment between these two points in the ratio $1: 2$.
(1997, 5M)
Match the List
Show Answer
Solution:
- Let $y=4 x+c$ meets $x y=1$ at two points $A$ and $B$.
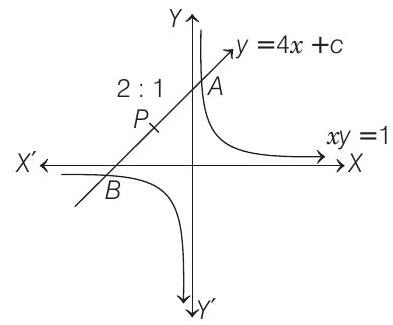
i.e. $A\left(t _1, 1 / t _1\right), B\left(t _2, 1 / t _2\right)$ $\therefore$ Coordinates of $P$ are
$$ \frac{2 t _1+t _2}{2+1}, \frac{2 \cdot \frac{1}{t _1}+1 \cdot \frac{1}{t _2}}{2+1}=(h, k) $$
$\therefore h=\frac{2 t _1+t _2}{3}$ and $k=\frac{2 t _2+t _1}{3 t _1 t _2}$
Also, $t _1, \frac{1}{t _1}$ and $t _2, \frac{1}{t _2}$ lie on $y=4 x+c$.
$\Rightarrow \quad \frac{\frac{1}{t _2}-\frac{1}{t _1}}{t _2-t _1}=\frac{1}{t _1 t _2}=4 \quad$ or $\quad t _1 t _2=-1 / 4$
From Eq. (i), $t _1=2 h+\frac{k}{4}$
$$ \text { and } \quad t _1=h-\frac{k}{2} $$
From Eqs. (ii) and (iii), $-h-\frac{k}{2} \quad 2 h+\frac{k}{4}=-\frac{1}{4}$
$$ \begin{array}{lll} \Rightarrow & -\frac{2 h+k}{2} \quad \frac{8 h+k}{4}=-\frac{1}{4} \\ \Rightarrow & (2 h+k)(8 h+k)=2 \\ \Rightarrow & 16 h^{2}+k^{2}+10 h k=2 \end{array} $$
Hence, required locus is $16 x^{2}+y^{2}+10 x y=2$.
