Functions 2 Question 14
15. Let
(1989, 2M)
(a)
(b)
(c)
(d)
Show Answer
Answer:
Correct Answer: 15.
Solution:
- Since, area of equilateral triangle
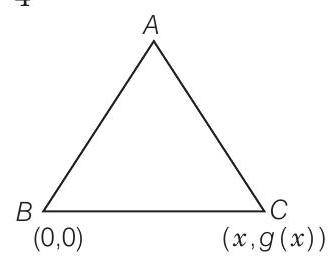
Hence, (b) and (c) are the correct options.






