Ellipse 1 Question 11
12. Let
Passage Type Questions
Passage
Let
(2016 Adv.)
Show Answer
Answer:
Correct Answer: 12. (a)
Solution:
- Given,
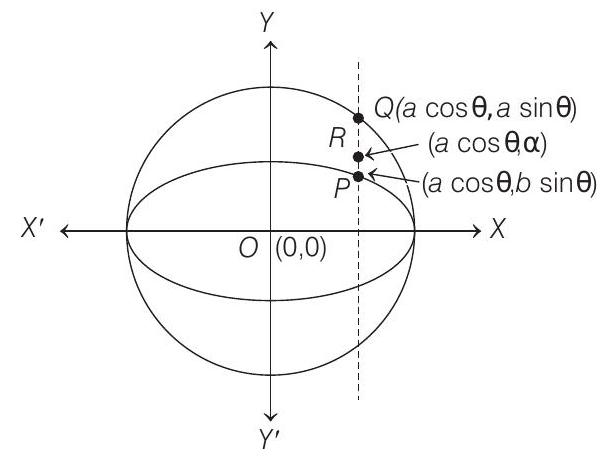
Let the coordinates of
and
On squaring and adding Eqs. (i) and (ii), we get
Hence, locus of






