Differential Equations 1 Question 12
12. Let $y(x)$ be a solution of the differential equation $\left(1+e^{x}\right) y^{\prime}+y e^{x}=1$. If $y(0)=2$, then which of the following statement(s) is/are true?
(2015 Adv.)
(a) $y(-4)=0$
(b) $y(-2)=0$
(c) $y(x)$ has a critical point in the interval $(-1,0)$
(d) $y(x)$ has no critical point in the interval $(-1,0)$
Show Answer
Answer:
Correct Answer: 12. (a, c)
Solution:
- Here, $\left(1+e^{x}\right) y^{\prime}+y e^{x}=1$
$\Rightarrow \frac{d y}{d x}+e^{x} \cdot \frac{d y}{d x}+y e^{x}=1$
$\Rightarrow d y+e^{x} d y+y e^{x} d x=d x$
$\Rightarrow \quad d y+d\left(e^{x} y\right)=d x$
On integrating both sides, we get
$$ y+e^{x} y=x+C $$
$$ \begin{aligned} & \text { Given, } \quad y(0)=2 \\ & \Rightarrow \quad 2+e^{0} \cdot 2=0+C \\ & \Rightarrow \quad C=4 \\ & \therefore \quad y\left(1+e^{x}\right)=x+4 \\ & \Rightarrow \quad y=\frac{x+4}{1+e^{x}} \\ & \text { Now at } x=-4, y=\frac{-4+4}{1+e^{-4}}=0 \\ & \therefore \quad y(-4)=0 \\ & \text { For critical points, } \quad \frac{d y}{d x}=0 \\ & \text { i.e. } \quad \frac{d y}{d x}=\frac{\left(1+e^{x}\right) \cdot 1-(x+4) e^{x}}{\left(1+e^{x}\right)^{2}}=0 \\ & \Rightarrow \quad e^{x}(x+3)-1=0 \\ & \text { or } \quad e^{-x}=(x+3) \end{aligned} $$
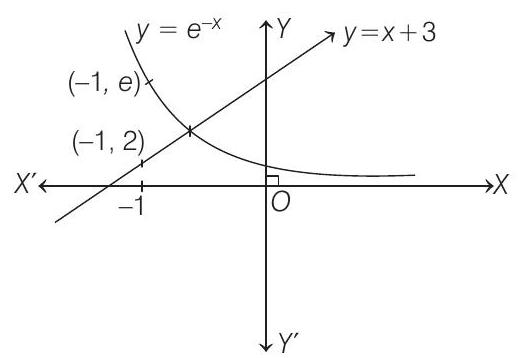
Clearly, the intersection point lies between $(-1,0)$.
$\therefore y(x)$ has a critical point in the interval $(-1,0)$.
