Complex Numbers 4 Question 6
6. The complex numbers
(a) of area zero
(b) right angled isosceles
(c) equilateral
(d) obtuse angled isosceles
Objective Questions II
(One or more than one correct option)
Show Answer
Answer:
Correct Answer: 6. (c)
Solution:
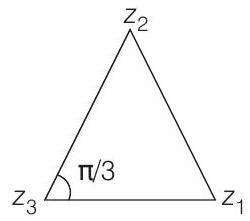
Hence, the triangle is an equilateral.
Alternate Solution
Therefore, triangle is equilateral.






