Complex Numbers 4 Question 16
16. Show that the area of the triangle on the argand diagram formed by the complex number $z, i z$ and $z+i z$ is $\frac{1}{2}|z|^{2}$.
(1986, $2 \frac{1}{2}$ M)
Show Answer
Solution:
- We have, $i z=z e^{i \pi / 2}$. This implies that $i z$ is the vector obtained by rotating vector $z$ in anti-clockwise direction through $90^{\circ}$. Therefore, $O A \perp A B$. So,
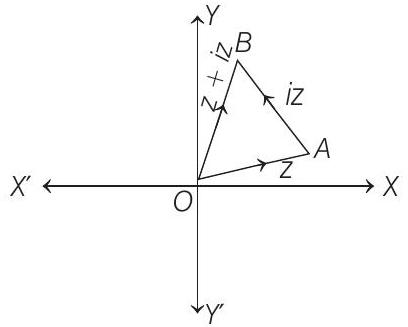
Area of $\triangle O A B=\frac{1}{2} O A \times O B=\frac{1}{2}|z||i z|=\frac{1}{2}|z|^{2}$
