Complex Numbers 4 Question 12
12. If one of the vertices of the square circumscribing the circle $|z-1|=\sqrt{2}$ is $2+\sqrt{3} i$. Find the other vertices of square.
(2005, 4M)
Show Answer
Answer:
Correct Answer: 12. $z _2=-\sqrt{3} i, z _3=(1-\sqrt{3})+i$ and $z _4=(1+\sqrt{3})-i$
Solution:
- Here, centre of circle is $(1,0)$ is also the mid-point of diagonals of square
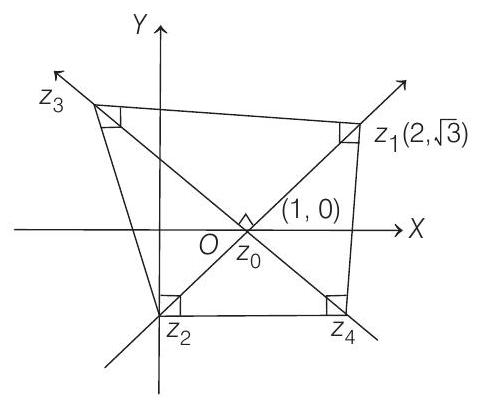
$$ \begin{array}{lc} \Rightarrow & \frac{z _1+z _2}{2}=z _0 \\ \Rightarrow & \left.z _2=-\sqrt{3} i \quad \text { [where, } z _0=1+0 i\right] \\ \text { and } & \frac{z _3-1}{z _1-1}=e^{ \pm i \pi / 2} \\ \Rightarrow & z _3=1+(1+\sqrt{3} i) \cdot \cos \frac{\pi}{2} \pm i \sin \frac{\pi}{2}\left[\because z _1=2+\sqrt{3} i\right] \\ & =1 \pm i(1+\sqrt{3} i)=(1 \mp \sqrt{3}) \pm i=(1-\sqrt{3})+i \end{array} $$
and $z _4=(1+\sqrt{3})-i$
