Complex Numbers 4 Question 10
10.
(1993, 2M)
Show Answer
Answer:
Correct Answer: 10.
Solution:
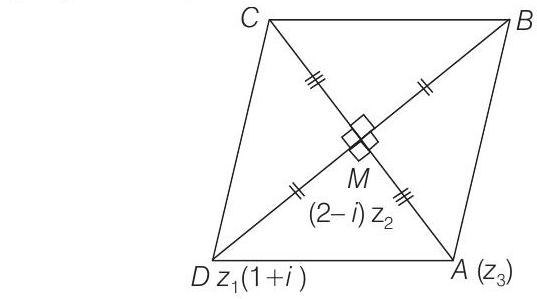
- Given,
and diagonals of a rhombus bisect each other.
Let
Again,
But
and
Now, let coordinate of
But in a rhombus
Again,
On putting these values in Eq. (i), we get
Therefore,
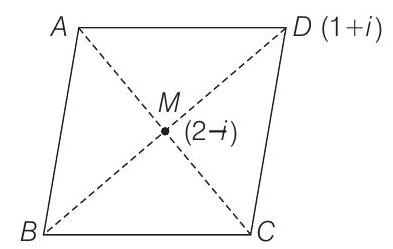
Alternate Solution
Since,