Complex Numbers 2 Question 7
7. If
(a) is equal to
(2014 Main)
(b) lies in the interval
(c) is strictly greater than
(d) is strictly greater than
Show Answer
Answer:
Correct Answer: 7. (b)
Solution:
Minimum
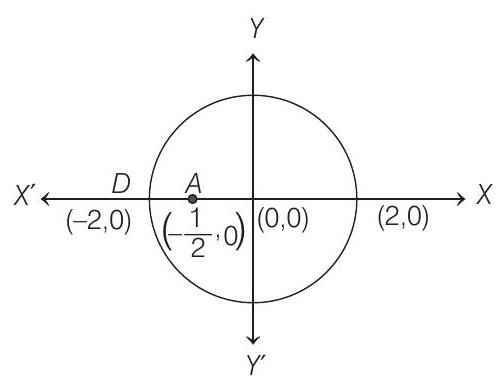
Geometrically Min
Hence, minimum value of






