Circle 5 Question 18
18.
Show Answer
Solution:
- Let the coordinate of point
We have,
Since,
Circle 415
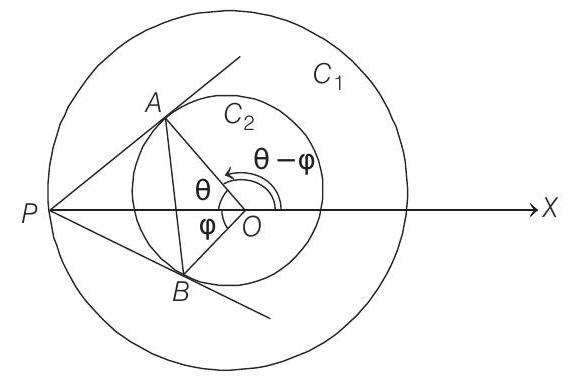
and that of
If
and
Now,






