Circle 4 Question 1
1. The locus of the centres of the circles, which touch the circle, $x^{2}+y^{2}=1$ externally, also touch the $Y$-axis and lie in the first quadrant, is
(2019 Main, 10 April II)
(a) $y=\sqrt{1+2 x}, x \geq 0$
(b) $y=\sqrt{1+4 x}, x \geq 0$
(c) $x=\sqrt{1+2 y}, y \geq 0$
(d) $x=\sqrt{1+4 y}, y \geq 0$
Show Answer
Answer:
Correct Answer: 1. (a)
Solution:
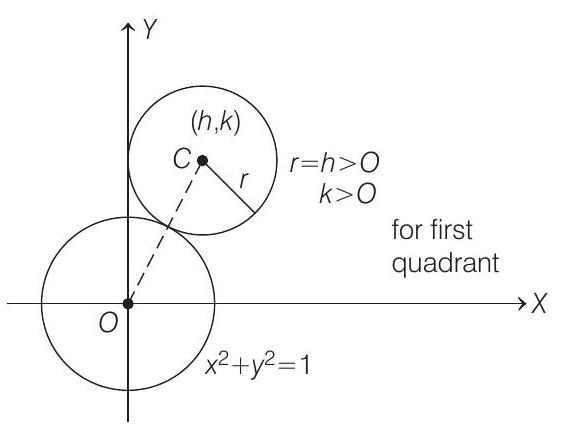
- Let $(h, k)$ be the centre of the circle and radius $r=h$, as circle touch the $Y$-axis and other circle $x^{2}+y^{2}=1$ whose centre $(0,0)$ and radius is 1.
$\therefore O C=r+1$
$[\because$ if circles touch each other externally, then $\left.C _1 C _2=r _1+r _2\right]$
$\Rightarrow \sqrt{h^{2}+k^{2}}=h+1, h>0$
and $k>0$, for first quadrant.
$\Rightarrow \quad h^{2}+k^{2}=h^{2}+2 h+1$
$\Rightarrow \quad k^{2}=2 h+1$
$\Rightarrow \quad k=\sqrt{1+2 h}$, as $k>0$
Now, on taking locus of centre $(h, k)$, we get
$y=\sqrt{1+2 x}, x \geq 0$
