Circle 2 Question 10
11. The $\triangle P Q R$ is inscribed in the circle $x^{2}+y^{2}=25$. If $Q$ and $R$ have coordinates $(3,4)$ and $(-4,3)$ respectively, then $\angle Q P R$ is equal to
$(2000,2 M)$
(a) $\pi / 2$
(b) $\pi / 3$
(c) $\pi / 4$
(d) $\pi / 6$
Show Answer
Answer:
Correct Answer: 11. (a)
Solution:
- Let $O$ is the point at centre and $P$ is the point at circumference. Therefore, angle $Q O R$ is double the angle $Q P R$. So, it is sufficient to find the angle $Q O R$.
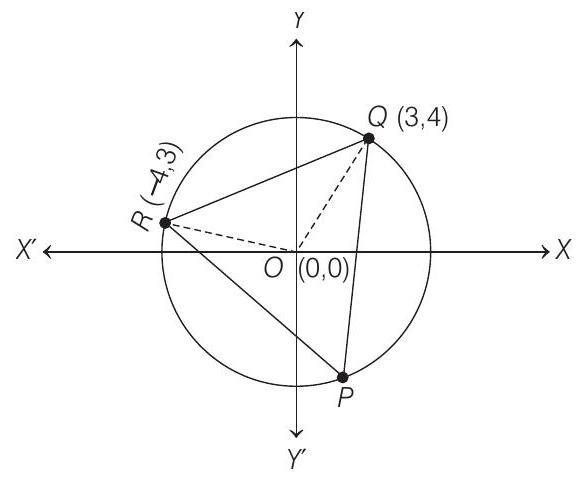
Now, slope of $O Q, m _1=4 / 3$,
slope of $O R, m _2=-3 / 4$
Here,
$m _1 m _2=-1$
Therefore, $\quad \angle Q O R=\pi / 2$
which implies that $\angle Q P R=\pi / 4$
