Circle 1 Question 24
24. Let $E _1 E _2$ and $F _1 F _2$ be the chords of $S$ passing through the point $P _0(1,1)$ and parallel to the $X$-axis and the $Y$-axis, respectively. Let $G _1 G _2$ be the chord of $S$ passing through $P _0$ and having slope -1 . Let the tangents to $S$ at $E _1$ and $E _2$ meet at $E _3$, then tangents to $S$ at $F _1$ and $F _2$ meet at $F _3$, and the tangents to $S$ at $G _1$ and $G _2$ meet at $G _3$. Then, the points $E _3, F _3$ and $G _3$ lie on the curve
(a) $x+y=4$
(b) $(x-4)^{2}+(y-4)^{2}=16$
(c) $(x-4)(y-4)=4$
(d) $x y=4$
Show Answer
Solution:
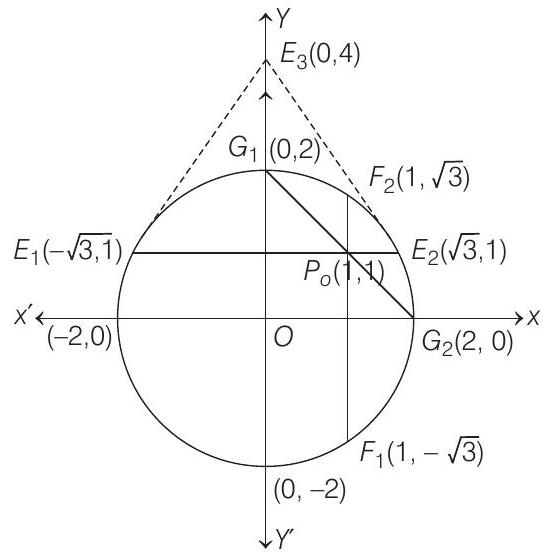
Equation of tangent at $E _1(-\sqrt{3}, 1)$ is
$$ \begin{aligned} -\sqrt{3} x+y & =4 \text { and at } E _2(\sqrt{3}, 1) \text { is } \\ \sqrt{3} x+y & =4 \end{aligned} $$
Intersection point of tangent at $E _1$ and $E _2$ is $(0,4)$.
$\therefore \quad$ Coordinates of $E _3$ is $(0,4)$
Similarly, equation of tangent at $F _1(1,-\sqrt{3})$ and $F _2(1, \sqrt{3})$ are $x-\sqrt{3} y=4$ and $x+\sqrt{3} y=4$, respectively and intersection point is $(4,0)$, i.e., $F _3(4,0)$ and equation of tangent at $G _1(0,2)$ and $G _2(2,0)$ are $2 y=4$ and $2 x=4$, respectively and intersection point is $(2,2)$ i.e., $G _3(2,2)$. Point $E _3(0,4), F _3(4,0)$ and $G _3(2,2)$ satisfies the line $x+y=4$.
