Application of Derivatives 4 Question 41
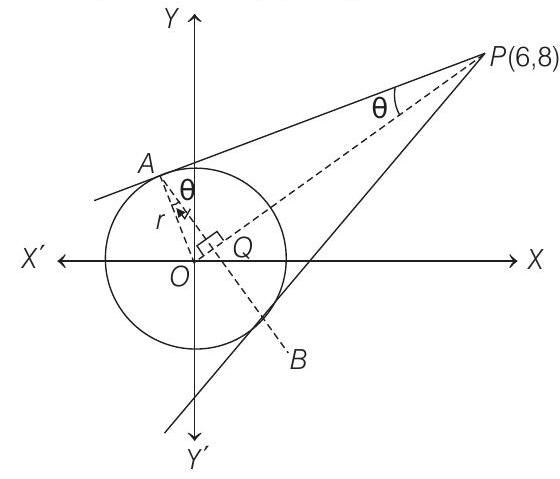
43. For the circle $x^{2}+y^{2}=r^{2}$, find the value of $r$ for which the area enclosed by the tangents drawn from the point $P(6,8)$ to the circle and the chord of contact is maximum.
(2003, 2M)
Show Answer
Answer:
Correct Answer: 43. Maxima at $x=\frac{\left(b-\sqrt{b^{2}-1}\right)}{4}$ and minima at $x=\frac{1}{4}\left(b+\sqrt{b^{2}-1}\right)$
Solution:
- To maximise area of $\triangle A P B$, we know that, $O P=10$ and $\sin \theta=r / 10$, where $\theta \in(0, \pi / 2)$
$\therefore \quad$ Area $=\frac{1}{2}(2 A Q)(P Q)$
$$ \begin{aligned} & =A Q \cdot P Q=(r \cos \theta)(10-O Q) \\ & =(r \cos \theta)(10-r \sin \theta) \\ & =10 \sin \theta \cos \theta\left(10-10 \sin ^{2} \theta\right) \quad \text { [from Eq. (i)] } \end{aligned} $$
$\Rightarrow \quad A=100 \cos ^{3} \theta \sin \theta$
$\Rightarrow \quad \frac{d A}{d \theta}=100 \cos ^{4} \theta-300 \cos ^{2} \theta \cdot \sin ^{2} \theta$
Put $\frac{d A}{d \theta}=0$
$\Rightarrow \cos ^{2} \theta=3 \sin ^{2} \theta$
$\Rightarrow \quad \tan \theta=1 / \sqrt{3}$
$\Rightarrow \theta=\pi / 6$
At which $\frac{d A}{d \theta}<0$, thus when $\theta=\pi / 6$, area is maximum
From Eq. (i), $r=10 \sin \frac{\pi}{6}=5$ units
