Application of Derivatives 4 Question 36
38. A line
Match List I with List II and select the correct answer using the codes given below the list.
| Column I | Column II | ||
|---|---|---|---|
| P. | 1. | ||
| Q. | Maximum area | 2. | 4 |
| of |
Codes
| (a) | 4 | 1 | 2 | 3 |
| (b) | 3 | 4 | 1 | 2 |
| (c) | 1 | 3 | 2 | 4 |
| (d) | 1 | 3 | 4 | 2 |
Passage Based Problems
Consider the function
Show Answer
Answer:
Correct Answer: 38. (a)
Solution:
- Here,
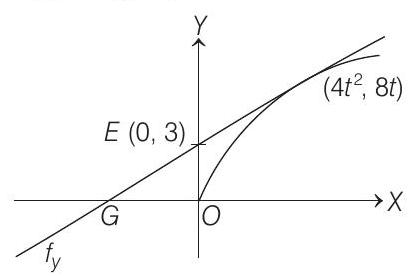
Tangent at
At
Also,






