3D Geometry 3 Question 35
35. Let $P$ be the image of the point $(3,1,7)$ with respect to the plane $x-y+z=3$. Then, the equation of the plane passing through $P$ and containing the straight line $\frac{x}{1}=\frac{y}{2}=\frac{z}{1}$ is
(2016 Adv.)
(a) $x+y-3 z=0$
(b) $3 x+z=0$
(c) $x-4 y+7 z=0$
(d) $2 x-y=0$
Show Answer
Answer:
Correct Answer: 35. (a)
Solution:
- Let image of $Q(3,1,7)$ w.r.t. $x-y+z=3$ be $P(\alpha, \beta, \gamma)$.
$ \begin{aligned} & \therefore \quad \frac{\alpha-3}{1}=\frac{\beta-1}{-1}=\frac{\gamma-7}{1}=\frac{-2(3-1+7-3)}{1^{2}+(-1)^{2}+(1)^{2}} \\ & \Rightarrow \quad \alpha-3=1-\beta=\gamma-7=-4 \\ & \therefore \quad \alpha=-1, \beta=5, \gamma=3 \end{aligned} $
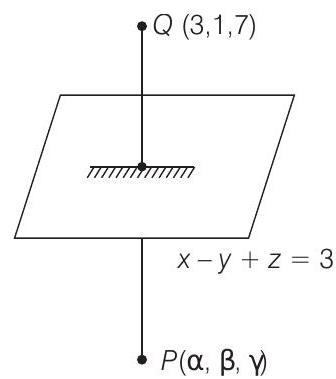
Hence, the image of $Q(3,1,7)$ is $P(-1,5,3)$.
To find equation of plane passing through $P(-1,5,3)$
and
containing $\frac{x}{1}=\frac{y}{2}=\frac{z}{1}$
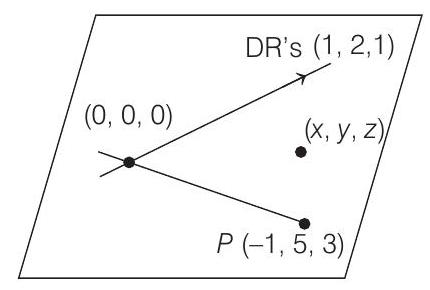
$\Rightarrow \quad\left|\begin{array}{cccc}x-0 & y-0 & z-0 \\ 1-0 & 2-0 & 1-0 \\ -1-0 & 5-0 & 3-0\end{array}\right|=0$
$ \begin{array}{lrl} \Rightarrow & x(6-5)-y(3+1)+z(5+2) & =0 \\ \therefore & x-4 y+7 z & =0 \end{array} $






